Math 431 Section 3 Practice Midterm 1 Fall 1998

    1. Solve the initial value problem

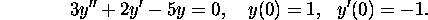
      displaymath298

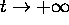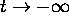    2. Determine the behavior of the solution as tex2html_wrap_inline308 and as tex2html_wrap_inline310 .
    3. Solve the differential equation

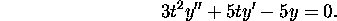
      displaymath299

      Hint: use substitution x=ln(t).

  2. Solve the differential equation

    displaymath300

    1. Solve the initial value problem

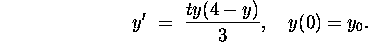
      displaymath301

    2. Determine how the behavior of the solution as t increases depends on the initial condition.
  4. Solve the differential equation

    displaymath302

    1. Solve the initial value problem

        eqnarray210

    2. How does the behavior of the solution as tex2html_wrap_inline316 depends on the initial condition?
    3. Classify points tex2html_wrap_inline318 in the plane according to the number of solutions of (1) passing through the the point tex2html_wrap_inline318 .
  6. Let p(t), q(t) be two continuous functions on tex2html_wrap_inline326 . Let tex2html_wrap_inline328 and tex2html_wrap_inline330 be a solutions of y''+p(t)y'+q(t)y=0 satisfying

    eqnarray216

    1. Are the two solutions 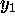tex2html_wrap_inline334 and tex2html_wrap_inline336 a fundamental set of solutions? Explain!
    2. Given that 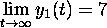tex2html_wrap_inline338 and tex2html_wrap_inline340 , what can you say about
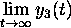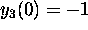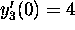      1. tex2html_wrap_inline342 if tex2html_wrap_inline344 is the solution satisfying tex2html_wrap_inline346 and tex2html_wrap_inline348 .
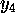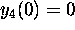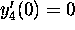      2. tex2html_wrap_inline350 if tex2html_wrap_inline352 is the solution satisfying tex2html_wrap_inline354 and tex2html_wrap_inline356 .