Math 621 Homework Assignment 2 Spring 2000

Due: Tuesday, February 22

1. Let

   

   Show that the real and imaginary parts of f satisfy the Cauchy-Riemann equations at z=0. Is f holomorphic at z=0?

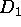2. 1. Let and 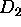be open connected sets in 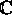and 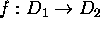a holomorphic map. Show that if H is harmonic in 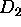, then the composition 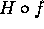is harmonic in .
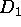   2. Let 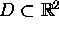be a connected open set and u(x,y), v(x,y) harmonic in D. Prove or disprove the following statements:
      1. The function 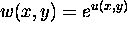is harmonic in D.
      2. The function w(x,y) = u(x,y)v(x,y) is harmonic in D.
      3. If v is a harmonic conjugate of u, the function 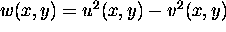is harmonic in D.
3. Ahlfors page 28 problem 3: Find the most general harmonic homogeneous polynomial of degree 3 (of the form ). Determine, by integration, the conjugate harmonic function and the corresponding analytic function (up to a constant).
4. Ahlfors page 28 problem 4: Show that if f is a holomorphic function with a constant absolute value , then f itself is a constant function.
5. 1. Prove that the functions f(z) and 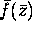are simultanously holomorphic (this is a special case of the Reflection Principle).
   2. Following Lang, we will say that a function f is analytic in an open set 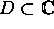, if for every point 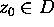, f has a power series expansion centered at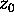

      

      with a positive radius of convergence. (We will see later that a function is analytic if and only if it is holomorphic, but do not assume it now). Prove that the functions f(z) is analytic in D if and only if 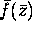is analytic in .

6. Lang page 58 problem 4a, c, d, g, h
7. Ahlfors, page 41 problem 4: If has radius of convergence R, what is the radius of convergence of ? of ?
8. Lang page 59 problem 10.
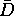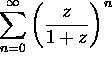9. Ahlfors, page 41 problem 8: For what values of z is convergent? (Describe the set geometrically).
10. Lang page 26 problem 7. Hint: Show first the following identity

    

11. Ahlfors, page 47 problem 8: Express in terms of the logarithm.
12. Ahlfors, page 47 problem 9: Use an appropriate branch of to define the angles of a triangle with vertices , bearing in mind that the angles should be between 0 and . With this definition, prove that the sum of the angles is .
13. Lang Ch. II Sec 3 page 68 problem 4.
14. Find a fractional linear transformation that maps
    1. to 1, -1, 0,
    2. 0, i, -i to 1, -1, 0.
15. Let . Determine the image of horizontal lines Im(z)=b under T. When the image is a circle, determine the center and radius.

Review point set topology in by reading

1. Lang, Ch I Section 4 pages 17-26 and
2. the Appendix ``Connectedness'' page 92-93 in Lang.

• About this document ...