NOTE
Here are the schedules for TWIGS Fall 2002 and
TWIGS Spring 2003.
Fall 2003 Schedule
Abstracts
26 September
Farshid Hajir, UMass Amherst
What Is A Newton Polygon?
Abstract
To a polynomial with rational coefficients and a prime p, we attach in a
simple way a polygon in the plane, its p-adic Newton Polygon, which
captures a surprisingly large amount of data concerning the roots of f.
I'll describe the origins of this concept in the work of Newton, its
development in the hands of Hensel and Dumas, and its applications to
algebraic questions such as factorization and calculation of Galois
groups.
3 October
Eric Sommers, UMass Amherst
What Is A Root System?
Abstract
A root system is a collection of vectors in a Euclidean space satisfying
certain axioms. I will present these axioms, describe the classification
of root systems, and explain some uses of root systems in Lie theory.
I'll finish with a discussion of some current problems that require
nothing more than the definition of a root system.
TOP
17 October
George Avrunin, UMass Amherst
What Is A Finite-State Verification?
Abstract
Finite-state verification is a collection of techniques that attempt
to answer the questions "Does a given computer system always do what
it is supposed to do?" and "Does it ever do what it's not supposed to
do?" Anyone who has used a large computer program knows that the easy
answers are almost certainly "no" and "yes", respectively; the hard
part is determining exactly how and under what circumstances the
system may misbehave. Finite-state verification techniques work by
constructing finite models that represent all the executions of the
system, and then apply various algorithms to check specific properties
of the system. I'll describe some of these techniques and the
interesting mathematical issues that arise in trying to make these
techniques practical for real systems.
TOP
7 November
Tom Braden, UMass Amherst
What (else) is a Newton Polytope?
Abstract
It has been known for a long time that "most" polynomials of
degree $n$ in one variable have $n$ distinct solutions
in $\mathbb C$. One can ask the same question for a system
of $d$ polynomials in $d$ variables. The notion of "degree"
is replaced by the Newton polytope, a convex region which controls
what monomials are allowed. The formula for the number of solutions
is given by the "mixed volume" of the Newton polytopes, a classical
quantity from convex geometry studied by Minkowski. If
time permits, we will also discuss another generalization
involving a single polynomial in several variables; since there
will be infinitely many solutions, the question must be rephrased
in terms of the "Euler characteristic", a topological invariant which
generalizes the size of a finite set of points.
TOP
7 November
Farshid Hajir, UMass Amherst
What Is An L-Function?
Abstract
An L-function is a way to package an arithmetic
function (a sequence of numbers) into an analytic object, whose
analytic properties (special values, residues, poles, analytic
continuation, functional equation, order of growth, ...) give the most
revealing portrait of the arithmetic data. The grand-daddy of all
L-functions is the Riemann Zeta Function. I will concentrate on the
case of elliptic curves, illustrating how L-functions act as an
analytic bridge between arithmetic/algebra and geometry.
TOP
14 November
Tom Weston, Amherst College
What Is A Motive?
Abstract
Roughly speaking, a motive is an arithmetic object coming from geometry.
Attempts to make that idea precise have frustrated mathematicians for the
forty years since Grothendieck initiated the subject. In this talk we
will focus on the reasonably concrete example of the motive of an
elliptic curve and its symmetric square. We will also hope to give some
idea of how the formalism of motives can be used to investigate
arithmetic questions.
TOP
21 November
Walter Rosenkrantz, UMass Amherst
What Is the Black-Scholes Option Pricing Formula?
Abstract
What is a fair price to pay for a financial asset with an
uncertain future return? We are particularly interested in determining
the fair price of an option, which is the right to buy (call option), or
sell (put option), a financial asset, e.g. stocks or bonds, at a fixed
price K called the strike price, by a certain date, called the
expiration date. The Black-Scholes options pricing formula solves this
problem via the concept of a ``replicating portfolio". The mathematical
derivation of this result is greatly simplified by first solving the problem
in the context of the ``single period binomial lattice Model". A straight
forward induction argument yields the options pricing formula for the
multi-period case, and a suitable application of the central limit theorem
yields the general result. In the course of deriving the Black-Scholes
options pricing formula we discuss the ``efficient markets hypothesis", the
random walk model for stock price fluctuations, and related questions of
independent interest.
TOP
21 November
Panos Kevrekides, UMass Amherst
What Is A Blowup?
Abstract
In this talk we'll discuss self-similar
blowup ("focusing") of waves in physical systems. As a benchmark
example of a PDE whose solutions display such behaviour, we will
examine the nonlinear Schr{\"o}dinger equation (NLS). We 'll review
the main wave solutions of the equation and their spectral properties,
revealing how they give rise to blowup as the relevant parameters are
varied. This effect will then be examined through a (very general)
dynamic renormalization point of view that allows one to observe and
justify the emergence of focusing solutions. The implications of such
a renormalization viewpoint on stability, the normal form of this
phenomenon, analogies of the self-similar blowup solutions with
travelling wave ones, and generalizations/extensions of this framework
will also be briefly discussed.
TOP
21 November
Paul Gunnells, UMass Amherst
What Is A Modular Form?
Abstract
TOP
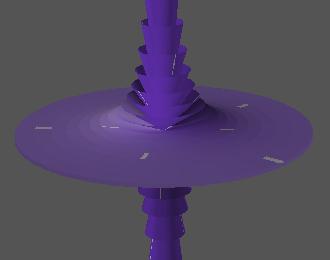
The picture above was created by
Paul Gunnells.
It visualizes the natural action of the group
of units of a complex cubic field on 3-space.
Consult
Paul for more details.
Last modified: 03 September 2003 by
Farshid Hajir

 The Seminar meets on most Fridays, 2:45-3:45
in 1634 LGRT.
The Seminar meets on most Fridays, 2:45-3:45
in 1634 LGRT.
 Driving Directions and
Campus Maps
Driving Directions and
Campus Maps
 Overview of the Seminar
Overview of the Seminar
