We'll show a few of these pictures of C_i. We must confess to a slight cheat: rather than using algebraic units coming from some number field F, I took sets of real vectors whose components satisfied the conditions of our theorem. (The problem is that with real units it's hard to get good pictures.)
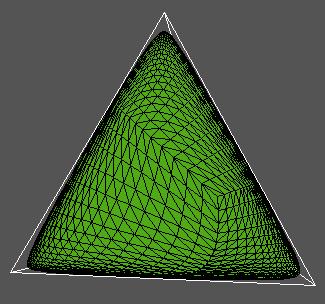
Figure 7 shows a small union of tetrahedra inside the big chamber.
Figure 7 |
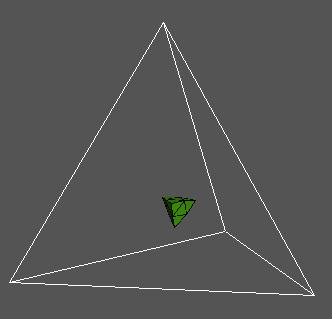
Figure 8 shows more tetrahedra ... now five along each side. It doesn't seem likely to eventually fill out the chamber in a nice way, since the corners appear headed for the faces of the chamber, but things will work out.
Figure 8 |
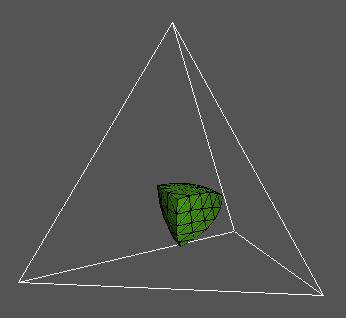
In figure 9 there are ten tetrahedra along a side. Note that the object seems to be inflating.
Figure 9 |
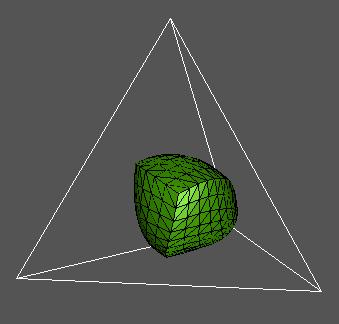
Now in figure 10 we start to see something nontrivial happening: the original corners are flattening out to become the faces of the object, and points inside the faces of the object are starting to head for the corners of chamber. In fact the units were chosen to force this to happen.
Figure 10 |
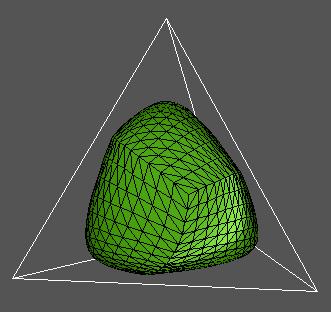
Finally the object is forty tetrahedra along a side, and we can see that the chamber is almost totally filled.
Figure 11 |