Complex cubic

Now X is C \times R. It's not so easy to actually get ones hands on C, so we content ourselves with getting some kind of picture of the action of U on X.

The unit group is a rank one abelian group, and the action of the fundamental unit basically shrinks along the R axis and stretches along the C plane, with a simultaneous irrational rotation about the R axis. If a unit has norm -1, then it interchanges the two halfspaces above and below C, whereas if it has norm 1 it preserves them. So as a first approximation to the picture one can look at the circular cones about the R axis generated by the units. That's what the following pictures show. Again most of the work was done by gp and geomview, but qhull was replaced by a few perl scripts to build the round cones, and then to assemble the final set of cones into a single object.

For a specific example, we can take the complex cubic field of discriminant -23. The generating polynomial is x^3-x^2+1, and any root of this polynomial is a fundamental unit u. It turns out that the norm of u is -1 (which is obvious from the polynomial). There are no interesting roots of unity, just +-1, so the unit group is generated by u.

Figure 4 shows an attempt to model the action of u. The R axis runs from left to right, and the viewer is sitting right on the C plane, The cones are given by taking a unit and rotatiing it about the R axis. The units actually sit at the end of the white segments.

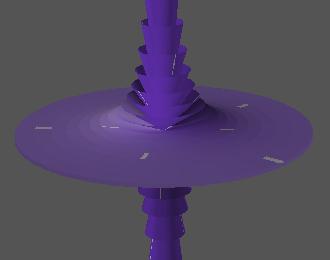
Figure 5 shows another view. We're now looking down the R axis onto the C plane. We can see that the cones get long and thin near R but flatten out to the plane C. The position of the white segments clearly shows the rotational aspect of the unit action (the segments only appear as rectangles because the cones obscure each other). If this field had more roots of unity then there would be more than one white segment per cone. Sadly this isn't the case.

Finally Figure 6 shows another view looking down at the C plane.